
Section 11. Macrosystems in equilibrium





11.1 Assumption about mechanical description
Gallavotti [Statistical Mechanics, a short treatise (Springer, 1999)] writes (p3), `the atomic hypothesis, apart from supposing the existence of atoms and molecules, assumes also that their motions are governed by a deterministic law of motion,’‘
Since atoms and molecules exist, their obeying mechanics cannot be a hypothesis but is a fact. However, is this really an empirically proved fact? The reader must reflect on
how to demonstrate that particles in a macroscopic system obey mechanics, whatever it is.
As noted in the text, the usual argument is to assume a certain mechanics and if every empirical result can be successfully explained, the assumption is vindicated. However, statistical mechanics never requires the whole of mechanics, so this usual argument cannot demonstrate the correctness of mechanics. [Besides, needless to say, logically, and practically, the usual strategy is not very desirable; it is only a self-consistency argument, so it cannot say anything about the question whether mechanics is really required or not. Also if we observe the world with prejudice, we tend to collect only facts we wish to observe.








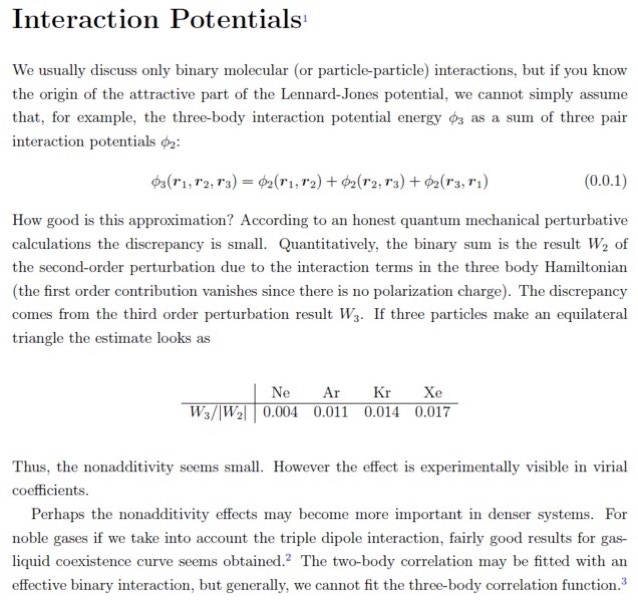
11.4 Interaction potential: how realistic is the binary interaction?
T Kihara’s Molecular forces tells us:









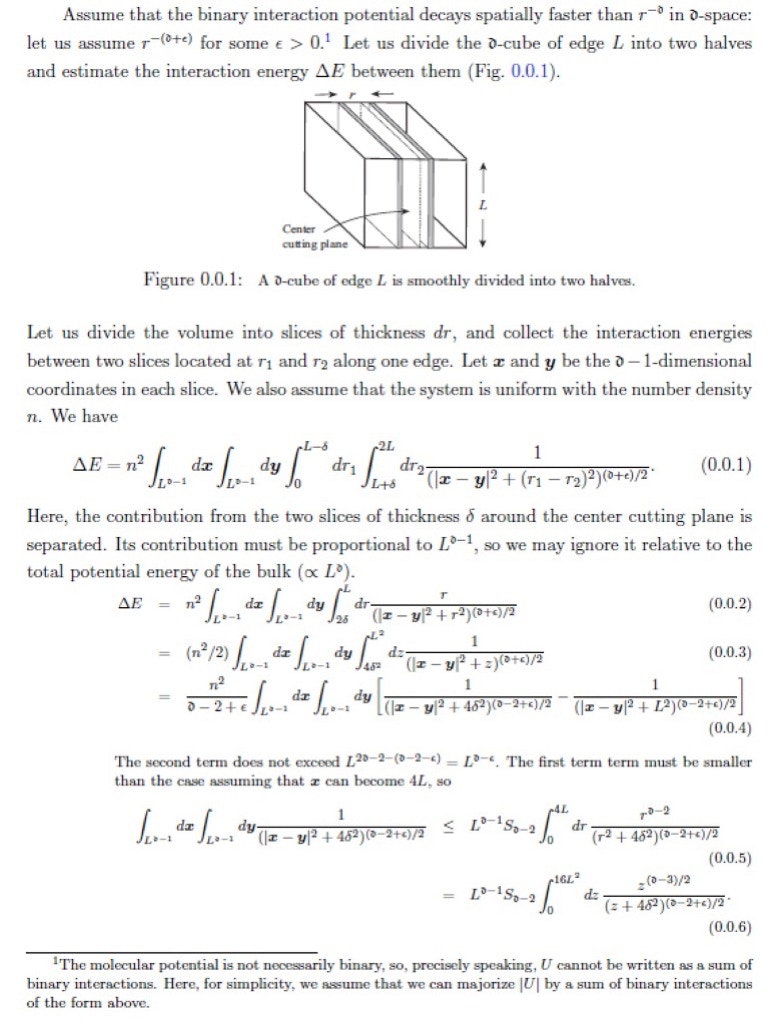
11.4 Additivity proof, an outline








11.8 Recurrence theorem 11.8 Recurrence.pdf








11.9 Elementary derivation of the Boltzmann equation 11.9 BoltzmannIntroduction.pdf



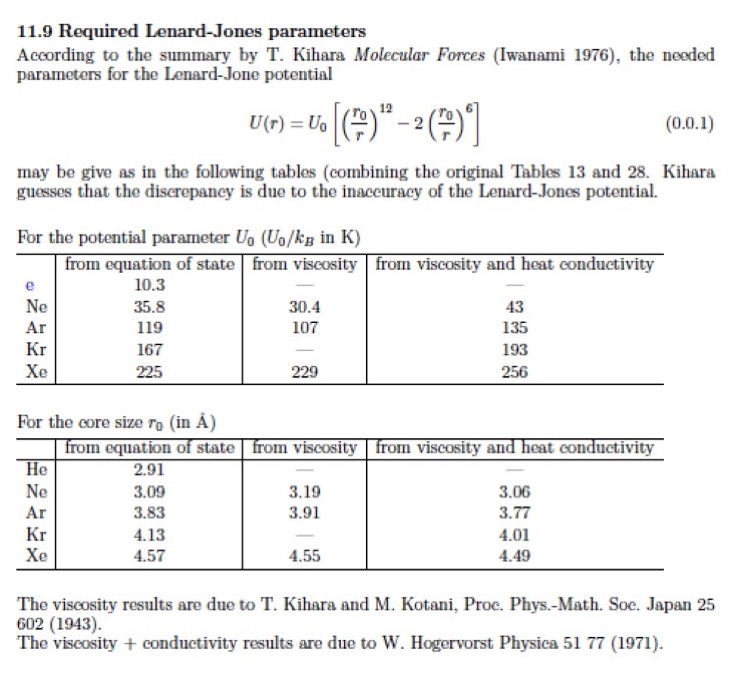





11.10 On the toy model illustrated in Fig. 11.2
Notice that the `long flat bottom’ (with occasional fluctuations) corresponds to the equilibrium state. We can learn several important lesson from the model.
(1) [As the purely deterministic model demonstrates] For almost all initial conditions, we very quickly reach the equilibrium state purely mechanically (deterministically). No external noise or disturbance is needed for the system to equilibrate. This corresponds to the `eigenstate thermalization’ in quantum mechanics. There is no doubt that pure mechanics can explain the approach of most systems to reach equilibrium.
(2) Furthermore, this approach has nothing to do with irreversibility. If the momenta are reversed, then the intial state will surely be recovered.
(3) However, if there is even a slightest external disturbance, it is extremely likely that the intial condition would never be recovered by time reversal. In this sense there is genuine irreversibility.
To recover a high peak in Fig. 11.2, we must `aim at the peak’ very accurately. That is, the `almost’ time reversed motion is required to recover the initial condition.
Why does time flow in the `positive’ direction and not in the opposite (or reversed direction)? First of all we must discuss how we determine the direction of time. If we wish to determine the direction of time, we must be able to determine the order of events we experience. This order must not be the order in time to avoid any circular argument, if we would not be contented with a self-consistency argument. Since we observe the world, our internal clock determines the direction of time. However, this clock is determined by certain reversible and irreversible physiological process. Therefore, our argument boils down to a kind of self-consistency argument.
A self-consistency argument is something like symmetry breaking: time ordering determines the ordering of events and the ordering of events supports the direction of time. We choose a certain direction as positive (according to e.g., our physiology).
This argument implies that the question why time flows only in one direction is a meaningless question (or no-science question), because in every possible world in which observers capable of conceiving natural science the direction of time conceived by the observer as `positive’ is defined as `positive.’


